Backtracking Algorithm 回溯算法

In a netshell 解题思路

In abraction, solving a backtracking problem is acutally iterating a decision tree. Every node of a tree has a valid answer and we traversing the tree to collect all the valid answers. 抽象地说，解决一个回溯问题，实际上就是遍历一棵决策树的过程，树的每个叶子节点存放着一个合法答案。你把整棵树遍历一遍，把叶子节点上的答案都收集起来，就能得到所有的合法答案。

To solve these problems, we need to consider:

1. Path：The decisions we have made.
2. Possible choices: The choices we can make now.
3. Ending condition: The bottom of the decision tree.

The framework of the backtracking algorithm:

• Make a choice before backtrack function and undo the choice after it.
• When reaching the ending condition, save the current path as the result.

results = []
def backtrack(path, choices):
    if ending_condition:
        results.add(path)
        return
    
    for choice in choices:
        # Make a choice
        backtrack(path, choice)
        # Undo the choice

Note: Typically backtracking algorithm does not have a way to pruning the decision tree, so the complexity is relative higher.

Two Examples 举个栗子

T46. Permutations 全排列

Given an array nums of distinct integers, return all the possible permutations. You can return the answer in any order.

Example 1:

Input: nums = [1,2,3]
Output: [[1,2,3],[1,3,2],[2,1,3],[2,3,1],[3,1,2],[3,2,1]]

Example 2:

Input: nums = [0,1]
Output: [[0,1],[1,0]]

Example 3:

Input: nums = [1]
Output: [[1]]

Constraints:

• 1 <= nums.length <= 6
• -10 <= nums[i] <= 10
• All the integers of nums are unique.

My solution

We can easily image the backtracking tree of this question. And the only thing need to do is traversing it to get the answer.

• Path：The number we have selected, saved in parameter track.

• Possible choices: The unused numbers, we use parameter used to save the used numbers and ignore them.

• Ending condition: The length of the path is the same as the number of numbers.

class Solution {

    List<List<Integer>> results = new LinkedList<>();

    public List<List<Integer>> permute(int[] nums) {
        boolean[] used = new boolean[nums.length]; //choices
        LinkedList<Integer> track = new LinkedList<>(); //path
        backtrack(nums, track, used);
        return results;
    }

    private void backtrack(int[] nums, LinkedList<Integer> track, boolean[] used) {
        int len = nums.length;
        
        //Ending conditions
        if (track.size() == len){
            results.add(new LinkedList(track));
        }
        
        for (int i=0; i<len; i++){
            if (used[i]){
                continue;
            }

            //Make a choice
            track.add(nums[i]);
            used[i] = true;

            backtrack(nums, track, used);

            //Undo a choice
            track.removeLast();
            used[i] = false;
        }
    }
}

T51. N-Queens N 皇后问题

The n-queens puzzle is the problem of placing n queens on an n x n chessboard such that no two queens attack each other.

Given an integer n, return all distinct solutions to the n-queens puzzle. You may return the answer in any order.

Each solution contains a distinct board configuration of the n-queens' placement, where 'Q' and '.' both indicate a queen and an empty space, respectively.

Example 1:

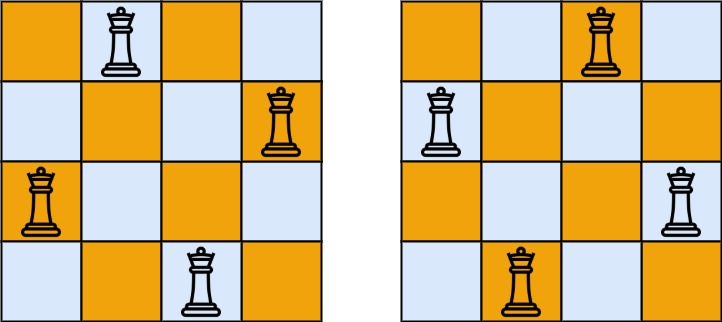
Input: n = 4
Output: [[".Q..","...Q","Q...","..Q."],["..Q.","Q...","...Q",".Q.."]]
Explanation: There exist two distinct solutions to the 4-queens puzzle as shown above

Example 2:

Input: n = 1
Output: [["Q"]]

Constraints:

• 1 <= n <= 9

My Solution

• Path：The position we have selected saved in board.

• Possible choices: The position follows the requirement, which is checked by function isValid()

• Ending condition: We reach the bottom row of the board

class Solution {
    List<List<String>> results = new LinkedList<>();

    public List<List<String>> solveNQueens(int n) {
        boolean[][] board = new boolean[n][n]; // Path
        backtrack(board, 0, n);
        return results;
    }

    private void backtrack(boolean[][] board, int row, int n) {

        //Ending condition
        if (row == n){
            List<String> result = new LinkedList<>();
            for (int i=0; i<n; i++){
                StringBuilder line = new StringBuilder();
                for (int j=0; j<n;j++){
                    line.append(board[i][j] ? "Q" : ".");
                }
                result.add(line.toString());
            }
            results.add(result);
        }

        for (int i=0; i<n; i++){
            if (!isValid(board, row, i, n)){
                continue;
            }
            //Make a choice
            board[row][i] = true;

            backtrack(board, row+1, n);

            //Undo a choice
            board[row][i] = false;
        }
    }

    private boolean isValid(boolean[][] board, int row, int column, int n){
        //Same column
        for (int i=0; i<row; i++){
            if (board[i][column]){
                return false;
            }
        }
        //left diagonal
        for (int i=row-1, j=column-1; i>=0 && j>=0; i--, j--){
            if (board[i][j]){
                return false;
            }
        }

        //right diagonal
        for (int i=row-1, j=column+1; i>=0 && j<n; i--, j++){
            if (board[i][j]){
                return false;
            }
        }
        return true;
    }
}